三角比で木の高さを求める
今回は、タンジェントを使って木の高さを測る問題を解説していきます。
タンジェントを使うことによって、下から木を眺めて高さを測ることができます。わざわざ登らなくても良いということですね!
↓三角比の定義はこちらをチェック!
木の高さをどうやって測る?
現代の便利なツールがないとしたら、実際にせっせと登って上からメジャーとかを垂らして測るのではないでしょうか?しかし、タンジェント(
高さ
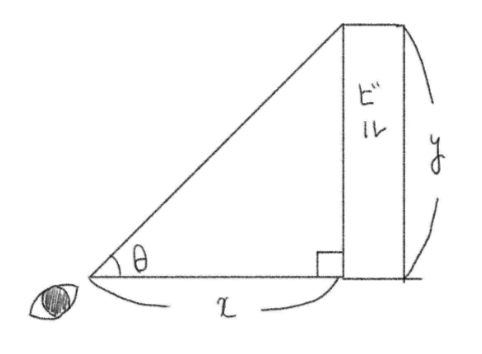
※図形問題のポイント
図形問題を解く際に、問題をただ眺めていても何をすれば良いのかは見えてきません。重要なことは、問題文から情報を抜き出し、図にしてみることです。そうすれば、何をすればいいのかが少しずつ見えてきます。
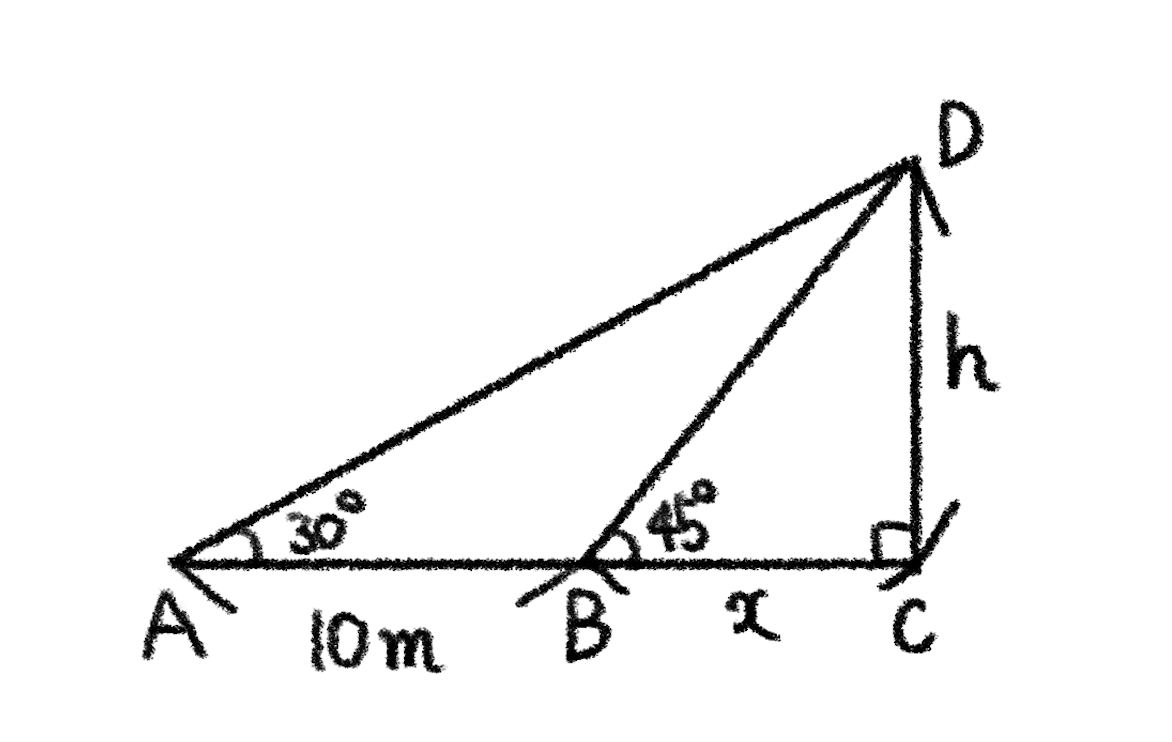
三角比で木の高さを求める(問題)
目の高さが
>>詳細はこちらから
三角比で木の高さを求める(答案の例)
よって、
①より
②より
木の高さを考慮すると、
三角比で木の高さを求める(解説)
図を描くための情報を抜き出しましょう。
- 木の前方の地点
これらの情報を図に落とし込むと、

※ 目の高さの
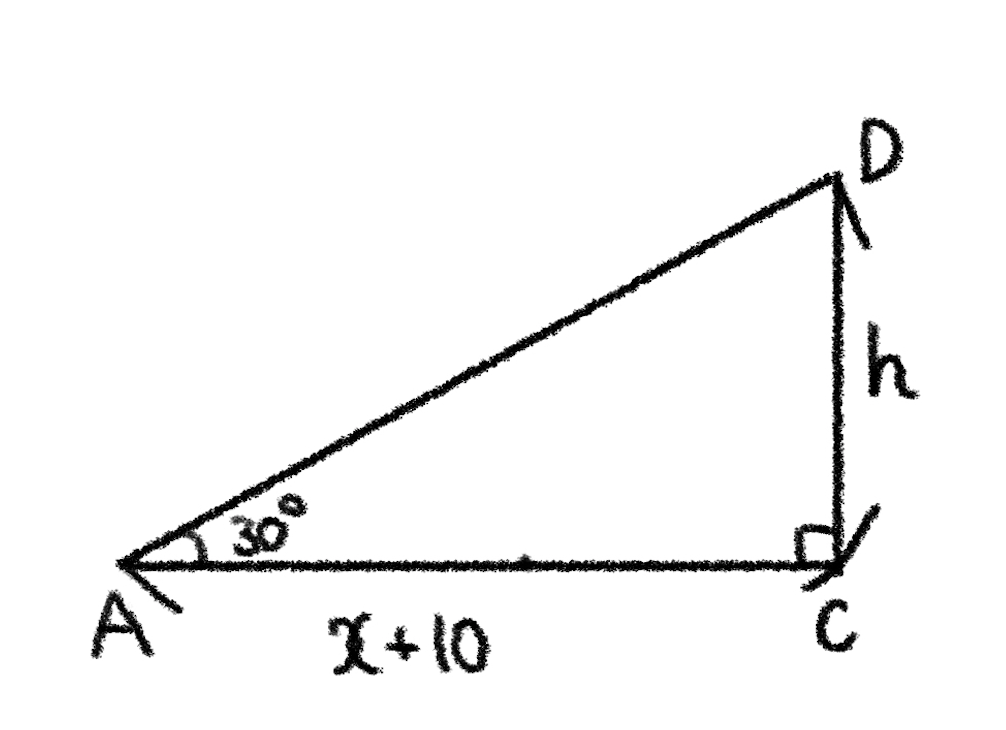
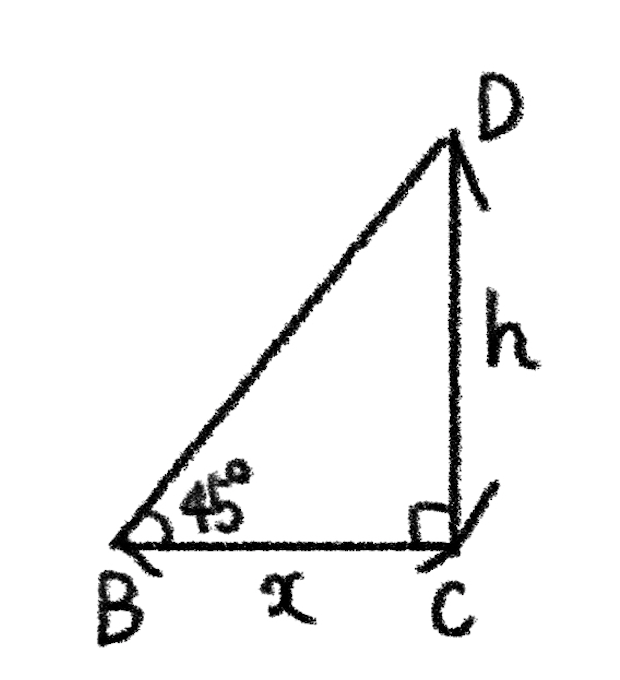
①より
②より
整理すると、
木の高さを考慮すると、
おわりに
今回は、タンジェントを使って木の高さを測る問題でした。
問題文を見て図にするのが難しかったかもしれません。
一度図にできれば、問題の糸口が見つけられるはずです。
さいごまで読んでいただきありがとうございました!
『統計の扉』で書いている記事
- 高校数学の解説
- 公務員試験の数学
- 統計学(統計検定2級レベル)
ぜひご覧ください!
数学でお困りの方は、コメントやXでご連絡ください。(Xはこちら)
私自身、数学が得意になれたのはただ運が良かったんだと思っています。たまたま親が通塾させることに積極的だったり、友達が入るって理由でそろばんに入れたり、他の科目が壊滅的だったおかげで数学が(相対的に)得意だと勘違いできたり。
”たまたま”得意になれたこの恩を、今数学の学習に困っている人に還元できたらなと思っています。お金は取りません。できる限り(何百人から連絡が来たら難しいかもですが…)真摯に向き合おうと思っていますのでオアシスだと思ってご連絡ください。



